All charged objects produce a thing we call their electric field. It is not observable in the conventional sense and might be thought of as a clever way to keep track of how charged objects interact. The field from one electron can influence another electron and the result is they feel a repelling force.
Large electric fields are in the atmosphere during storms.
Pre-lecture Study Resources
Watch the pre-lecture videos and read through the OpenStax text before doing the pre-lecture homework or attending class.
BoxSand Introduction
Electric Fields | Point Charges
Point Charge
The electric field for a point charges points away from positive charges and towards negative charges. It's magnitude has an inverse square dependency on the distance from the charge. If you double your distance from the charge the field decreases by a factor of 4. All of this information is contained in the expression below.
Electric Field for a Point Charge
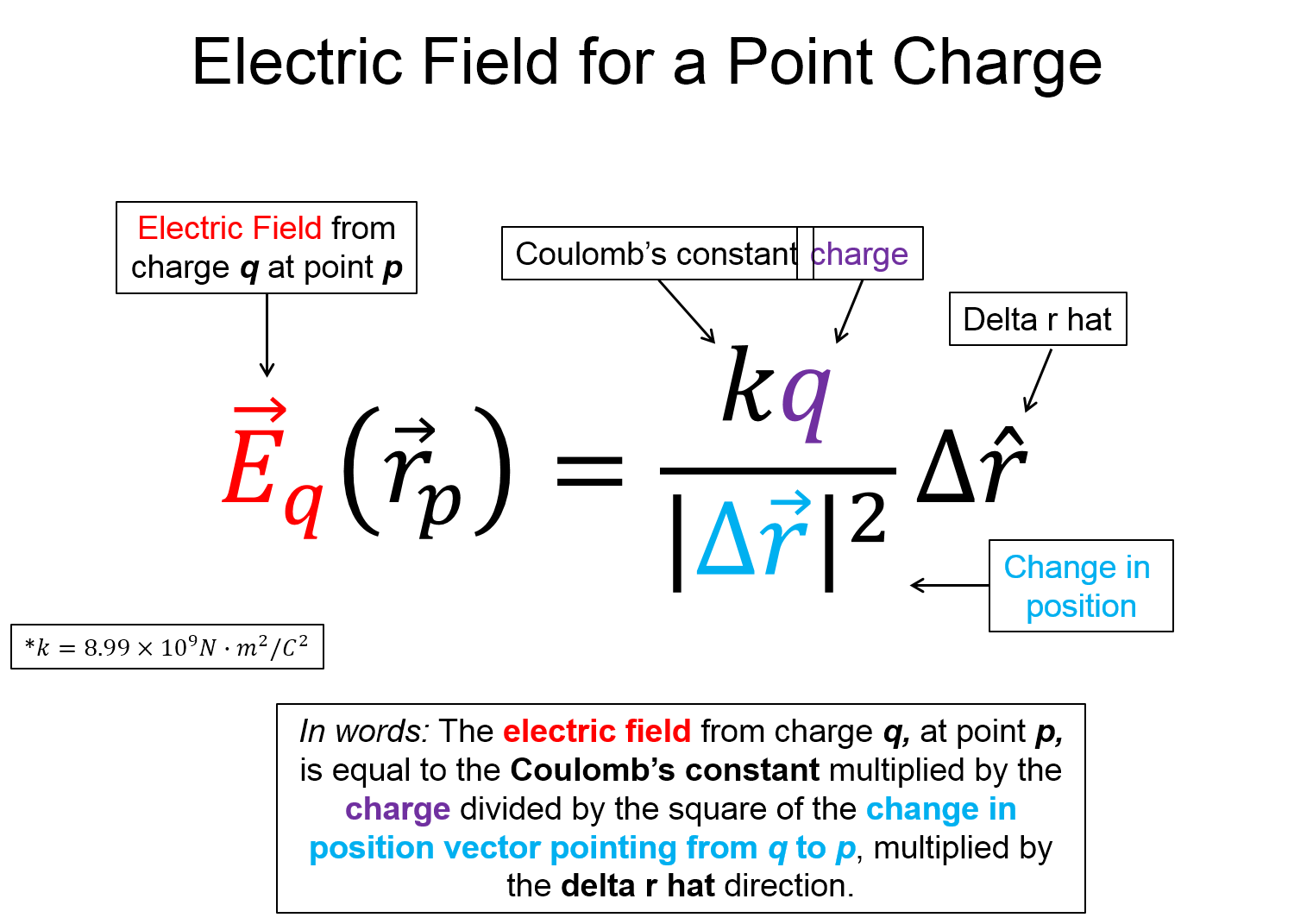
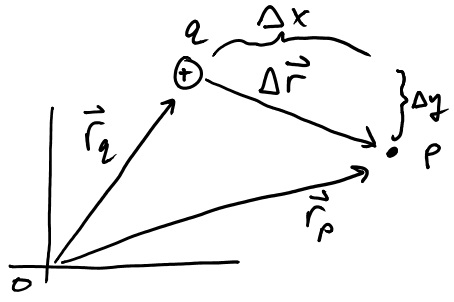 →Eq(→rp)=kq|Δ→r|2Δˆr
→Eq(→rp)=kq|Δ→r|2Δˆr
The above equation reads that the electric field from charge q, at point p, is equal to a constant (k=8.99x109Nm2/C2) times the charge, divided by the square of the absolute value of the change in position from q to p, multipled by the delta r hat direction. The Δˆr is a unit vector, meaning it has magnitude of 1 and only provides a direction.
Δ→r=<Δx,Δy,Δz>
Δˆr=<Δx|Δ→r|,Δy|Δ→r|,Δz|Δ→r|>
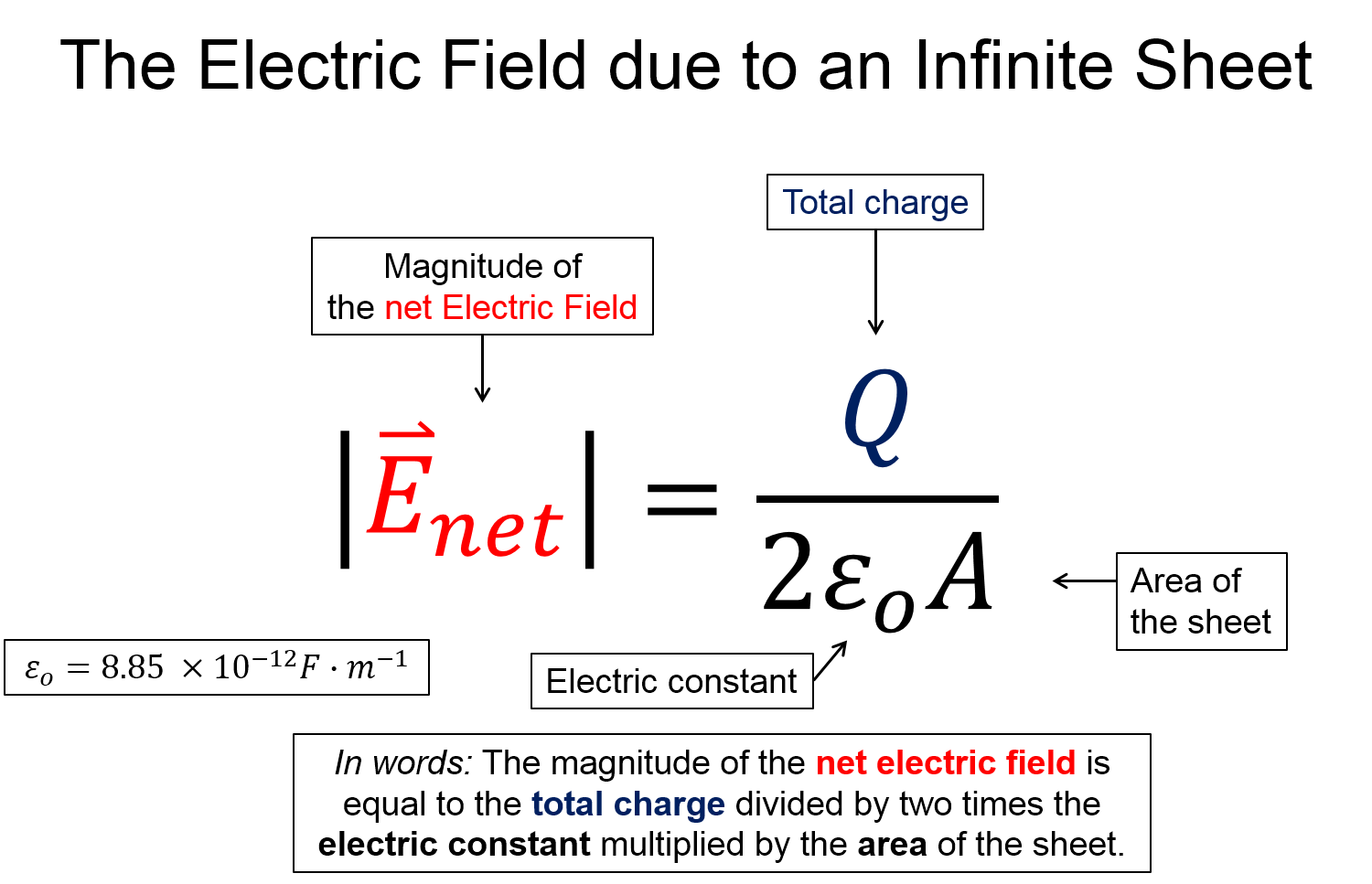
Key Equations and Infographics
Now, take a look at the pre-lecture reading and videos below.
BoxSand Videos
Required Videos
Suggested Supplemental Videos
OpenStax Reading
Fundamental examples
(1) What is the magnitude of the Electric field at a location r=2nm from a point charge with charge q=10nC that is located at the origin?
(2) Three point charges q1=q2=q3=5nC are placed equidistant from each other on the x-axis: q1 is located at x=−2nm, q2 is located at the origin, and q3 is located at x=2nm. A point charge q4=1nC is placed on the y-axis, 2 namometers up from the origin. (a) What is the magnitude of the electric force that q4 feels? (b) What is the direction of the electric force on q4? (c) What is the direction of the electric force if q4=−1nC instead?
(3) A point charge q1=−20nC is located at a position r1=<−3,0>nm. (a) What is the magnitude of the electric field at point ra=<0,3>nm. (b) By what factor does the magnitude of the electric field at point ra decrease if another point charge with magnitude q2=20nC is placed at the origin? (c) Calculate the magnitude and direction of the force that a point charge with magnitude q3=−1nC would feel if it were placed at position ra for both cases (a) and (b). [Optional (d) What is the electric force on q3 if charge q1 is removed?]
(4) (More time-consuming - practice with computing the electric field from multiple source charges in an arbitrary configuration) Three point charges q1=5nC, q2=10nC, and q3=−2nC are placed as follows: q1 is located at r1=<−3,0>nm, q2 is located at r2=<0,0>nm, and q3 is located at r3=<1,2>nm. (a) What is the magnitude and direction of the electric field at point $r_p = <8, 8> \hspace{0.2 cm}nm?
Solutions found HERE
Short foundation building questions, often used as clicker questions, can be found in the clicker questions repository for this subject.
Post-Lecture Study Resources
Use the supplemental resources below to support your post-lecture study.
Practice Problems
BoxSand practice problems - Answers
BoxSand's multiple select problems
BoxSand's quantitative problems
Recommended example practice problems
- *OpenStax, has practice problems at the end of every section
- Electric Field: Concept of a Field Revisited, Website Link
- Electric Field Lines, Website Link
- Conductors and Electric Fields, Website Link
- PhysicsClassroom, 27 problems on charge and the electric field, Website Link
- University of Greenbay: Guided problem on the electric force, Website Link
For additional practice problems and worked examples, visit the link below. If you've found example problems that you've used please help us out and submit them to the student contributed content section.
Additional Boxsand Study Resources
Additional BoxSand Study Resources
Learning Objectives
Summary
Summary
Atomistic Goals
Students will be able to...
YouTube Videos
Pre-Med Academy has a lot of videos about the Electric Field and Force, too many to list here. We really recommend checking out the content repository for this section and check out the rest of their videos Here are just a few on The Electric Field, Electric Field of a Point Charge, and Electric Field of an Infinite Wire.
Doc Schuster has a few good videos on the Electric field.
Other Resources
This link will take you to the repository of other content related resources .
Simulations
This flashphysics applet lets you create charge distributions from point charges and plot the corresponding electric field. Try placing test charges in the region of space to watch possible trajectories!
This PhET interactive is a test of skill! place charges to guide a charged hockey puck around obstacles and into the goal
This Puzzle by Duffy will help you connect the charge and the field.
The Puzzle by Duffy is a bit more challenging.
Use this 3D applet by Falstad to visualize the field for various charge distributions.
For additional simulations on this subject, visit the simulations repository.
Demos
History
Oh no, we haven't been able to write up a history overview for this topic. If you'd like to contribute, contact the director of BoxSand, KC Walsh (walshke@oregonstate.edu).
Physics Fun
Other Resources
Use the supplemental resources below to support your post-lecture study.
Boston University's Page on electric Field is a neat reference with a couple of example problems
PPLATO is a complete resource with a lot of information, and several practice questions per subject. This webpage covers electric charge, the electric field, and electric potential, we've already covered charge, so focus on the electric field, we'll get to electric potential in the next section.
The Physics Classroom's section on electric fields.
Isaac Physics' section on the electric field is a good short resource on the electric field. On this page there are two drop down sections on electric potential, we'll get to those in the next section, so have a look at the Electric Field, Visualizing and adding electric fields and Electric Fields in Conductors sections
Other Resources
This link will take you to the repository of other content related resources .
Problem Solving Guide
Use the Tips and Tricks below to support your post-lecture study.
Assumptions
Checklist
Misconceptions & Mistakes
Pro Tips
Multiple Representations
Multiple Representations is the concept that a physical phenomena can be expressed in different ways.
Physical
Mathematical



Graphical
Descriptive
Experimental