What is light?
Pre-lecture Study Resources
Watch the pre-lecture videos and read through the OpenStax text before doing the pre-lecture homework or attending class.
BoxSand Introduction
Waves | Case Studies: Strings, Sound, and Light
A traveling wave is comprised of a bunch of individual oscillators moving in a seamingly cooperative way such that a new feature arises from the their collective motion.
-- Specific Systems --
The velocity for a wave on a string or rope depends on the tension $F^T$ and the mass per length $\mu$.
$v_{string}=\sqrt{\frac{F^T}{\mu}}$
The velocity for a sound wave in a gas depends on the adiabatic constant $\gamma$ (specific to each gas), the gas constant $R$, temperature $T$, and molar mass of the gas $m$. At standard temperature and pressure it has a value of 343 m/s.
$v_{gas}=\sqrt{\frac{\gamma R T}{m}}$
The velocity for light in a vacuum is a constant value.
$v_{light}= 2.998x10^8 m/s$
-- Waves on a boundary --
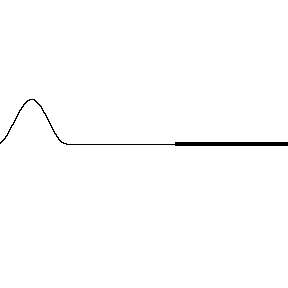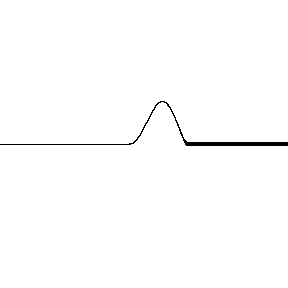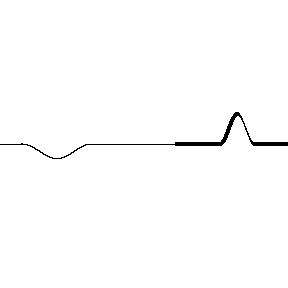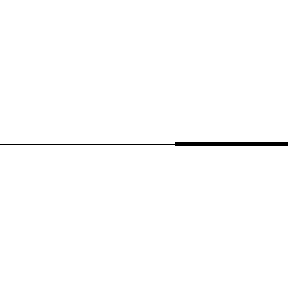
When a wave travels from one medium to another, like from a light string to a heavy string, the frequency of the wave on both sides of the boundary must be the same. One way to justify this claim is to imagine a point on both sides of the boundary of a light string tied to heavy string. If it's not going up and down at the same rate on both sides of the boundary, the knot holding the strings together will break. This is a way of stating the wave must be continous. Since the speed changes based on the features of the medium, the wavelength will change as well to satsify the relationship $v = f \lambda$.
 * Images: Dr. Russel
* Images: Dr. Russel 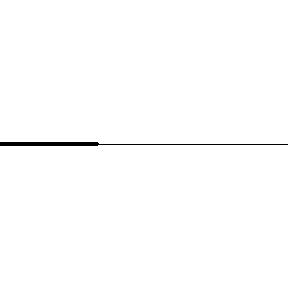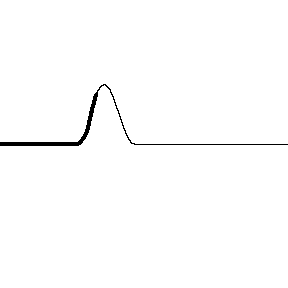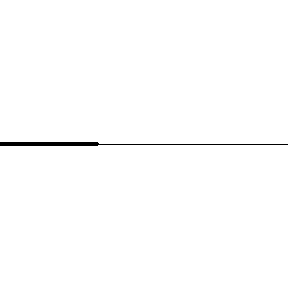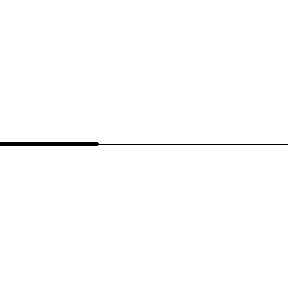
Key Equations and Infographics





Now, take a look at the pre-lecture reading and videos below.
BoxSand Videos
Required Videos
Suggested Supplemental Videos
OpenStax Reading
OpenStax Section 17.1 | Sound
OpenStax Section 17.2 | Speed of Sound, Frequency, and Wavelength
OpenStax Section 24.3 | The Electromagnetic Spectrum (supplemental)
Fundamental examples
(1) A traveling sound wave moves through a medium and the displacement can be described by the following function: $D(x,t) = (3 \mu m) sin (18x - 455t))$ where x is in meters and t is in seconds. Determine (a) the amplitude, (b) the wavelength, and (c) the speed of this wave.
(2) Consider a situation in which a light string is tied to a heavy string. The heavy string has a linear mass density that is three times as large as that of the light string. A 1-m-wavelength wave travels down the light string and into the heavy string. What is the wavelength of the wave in the heavy string?
(3)
Short foundation building questions, often used as clicker questions, can be found in the clicker questions repository for this subject.
Post-Lecture Study Resources
Use the supplemental resources below to support your post-lecture study.
Practice Problems
Conceptual problems
BoxSand's multiple select problems
BoxSand's quantitative problems
Recommended example practice problems
- The Physics Classroom, good set of problems Website Link
- Openstax's section on Traveling Waves has great practice problems, Website Link
For additional practice problems and worked examples, visit the link below. If you've found example problems that you've used please help us out and submit them to the student contributed content section.
Additional Boxsand Study Resources
Additional BoxSand Study Resources
Learning Objectives
Summary
Summary
Atomistic Goals
Students will be able to...
- 1. Be able to take a physical phenomena and represent it as a history graph or a snapshot graph.
- 2. Be able to identify distinguishing features of mechanical, E&M, and matter waves.
- 3. Be able to mathematically model a traveling wave using a function that has the form of $D(x,t)=D_{max} \sin(kx-\omega t)$ and be able to manipulate this equation to find relations between D, v and a.
- 4. Understand the connection between waves and oscillations.
- 5. Understand that the frequency of a wave is dependent only upon the source.
- 6. Understand that the speed of the wave depends on the medium in which it travels.
YouTube Videos
In this video Doc Schuster gives a thorough introduction to wave motion as a function of x and t.
Here is Doc Schuster giving a good run down of this section on Traveling Waves,
Other Resources
This link will take you to the repository of other content related resources .
Simulations
This link takes you to the PhET simulation for travelling waves.
You don't need Java to run this application.
For additional simulations on this subject, visit the simulations repository.
Demos
History
Oh no, we haven't been able to write up a history overview for this topic. If you'd like to contribute, contact the director of BoxSand, KC Walsh (walshke@oregonstate.edu).
Physics Fun
Other Resources
This site by Dan Russel at PSU uses a number of animations to explain the modes of waves.
The Physics classroom discusses the difference between standing waves and traveling waves.
Hyperphysics's concise reference for traveling waves.
Hyperphysics actually has several sections related to traveling waves. Using the concept map, click on the sections down the second path from the left.
| Waves Introduction | Transverse Waves |
| Longitudinal Waves | Wavelength and Frequency in Relation to Sound |
The University of Louisville Department of Physics has a nice overview page with great animations.
This web page has several animations showing the different types of waves, and covers the mathematical representation in a suscinct matter.
Other Resources
This link will take you to the repository of other content related resources .
Resource Repository
This link will take you to the repository of other content related resources.
Problem Solving Guide
Use the Tips and Tricks below to support your post-lecture study.
Assumptions
Remember that frequency is what determines a wave's energy, and as such cannot change as it travels since energy must be conserved. Instead, the wavelength, $\lambda$ is the thing we always assume changes as a wave travels through different mediums. Also since $v = \lambda f$, velocity will change as $\lambda$ changes.
It is also worth pointing out that period, $T$, and angular frequency, $\omega$ are related to frequency like so:
$T=\frac{1}{f}$ and $\omega = 2 \pi f$
As such, a wave's period and angular frequency are also properties of the wave itself and not the medium and will not change as the wave travels. Also since color is tied specifically to frequency, color will also not change as the wave enters different mediums.
Checklist
When solving a traveling wave problem you will most likely be using the wave equation $D(x,t) = Asin(kx - \omega t)$. Remember that $k = \frac{2 \pi}{\lambda}$ and that $\omega = 2 \pi f$ where $\lambda$ is the wavelength and $f$ is the frequency of the wave.
Graphical problems:
Draw a physical representation of your system and label any quantities given in the problem statement. It is a good idea to either draw a sketch of $x$ versus $t$ or an overhead snapshot of the situation, depending which is appropriate - for example, for the motion of a particle in a vibrating string, $x$ versus $t$ is more appropriate; for analyzing ripples on a pond radiating from a point, the overhead view will be more appropriate. Label wavelengths and/or periods.
Graphical problems will typically ask you about how many cycles will pass a point in a given amount of time, or how many wavelengths will fit in some area. Remember that a wavelength is a distance in meters that tells you how far a wave must travel to be at the same point in its cycle; the period is a time that tells you how long a wave must propogate for before it returns to the same point in their cycle.
Other problems:
Identify the type of wave - is it mechanical or electromagnetic? If it is an electromagnetic wave then you immediately know that its traveling with speed $ c = 3 * 10 ^ 8 $ m/s, and you can quickly find the wavelength if given the frequency, and vice-versa. Recall that $v_{wave} = f \lambda$. A common question when considering traveling waves is how long it takes a wave to travel from point A to point B. Understanding the wavespeed makes this problem easy - the distance is just $d = v t$.
All of the mathematical operations inside of the cosine/sine function can make the wave equation look intimidating, but are simple to handle case-by-case. For example, say you have the following equation - $D(x,t) = A sin( 30x - 50t) $ - and you are asked what are the wavelength and period of the wave. A good way to remember which terms are associated with wavelength and frequency is to inspect the equation and notice that there is an $x$, which we typically measure in meters, and $t$, which we measure for time. Since we want the argument inside of a trig function to be dimensionless, we know that $x$ is associated with wavelength and $t$ with the frequency or period.
Since the wave equation is $D(x,t) = Asin(kx - \omega t)$, we know that in the previous example
$$k = \frac{2 \pi}{\lambda}$$
$$⇒ \lambda = \frac{2 \pi}{30} = 0.21 m $$
and
$$ \omega = 2 \pi f $$
$$⇒ f = \frac{\omega}{2 \pi} = 8 s^{-1} $$
$$ T = \frac{1}{f} = 0.125 s $$
Misconceptions & Mistakes
- Mechanical traveling waves require a medium, but the particles of that medium do not travel with the wave: they propogate the wave by oscillating about some position.
- Electromagnetic waves are self-propogating; they do not require a medium and can travel through vacuum.
- The wave speed $v = \lambda \, f$ is not the speed of the oscillators, it is the speed of the feature of the wave itself. For example, a traveling wave on a string the oscillators are the tiny pieces of string that move up and down; the wave speed describes the speed of the crest that travels along the string, not the up and down motion.
- Not understanding the SHM nature of each particle in the wave.
- Speed of wave vs. speed of the particles in the wave.
- Use of degrees vs. radians for the function arguments.
- Direction of wave motion not discerned from equation; not connect to sinosoidal graph.
- Water waves are neither transverse or longitudinal waves, they are a combination of the two.
- The linear mass density of a string is the total mass divided by the total length. Experimentally a commom mistake is to measure the total mass and divide by the length between two fixed ends of the string when the string is clamped and ready to oscillate back and forth to observe traveling waves.
Pro Tips
- $v_{wave} = \lambda * f $ is a really useful equation to have in your back pocket, both for understanding and practicality. Remember that this equation means that the crest of a wave must travel a distance of one wavelength during one period. Practically speaking, this is sometimes a helpful equation to pull out when you have too many unknowns and need another equation.
Multiple Representations
Multiple Representations is the concept that a physical phenomena can be expressed in different ways.
Physical
There are two basic traveling waves: Logitudinal, Transverse. Traveling waves may be a combination of both types. Longitudinal waves travel in a line, whereas, transverse waves propogatae up and down.

Mathematical
$\nu = f \lambda$
$\kappa = \frac{2 \pi}{\lambda},\; \omega = \frac{2 \pi}{T}$
$\nu_{string} = \sqrt{\frac{F^{T}}{\mu}}$
$\nu_{sound} \propto \sqrt{Temperature}$
$x(t) = \pm x_{max} \frac{\sin \text{or}}{\cos} (\kappa x \pm \omega t)$
Velocity ($\nu$), Frequency ($f$), Wavelength ($\lambda$), Wave number ($\kappa$), Angular frequency ($\omega$),
Linear mass density ($\mu$), Force tenstion ($F^{T}$), Period ($T$)





Graphical
This is a snapshot graph that depicts a a wave at different points in time.

A history graph describes the history of a specific position with respect to time

Descriptive
Traveling waves are comprised of longitudinal and transverse waves. Longitudinal waves propogate in a line, whereas, transverse waves propogate up and down. Traveling waves may also be comprised of both types of of basic waves. For example, electromagnetic waves are a combination of both transverse and longitudinal traveling waves.
Experimental
Longitudinal and transverse waves experiments are easily performed using a slinkly for longitudinal waves or a rope for trasnverse waves. Check out the videos below to see the experiments.
Longitudinal
Transverse