It is easy to measure the electric potential using a volt meter. If you can map out the voltage in some region of space, you can determine the electric field from that voltage map. It is in this way that once you know the electric potential, you know everything.
Pre-lecture Study Resources
Watch the pre-lecture videos and read through the OpenStax text before doing the pre-lecture homework or attending class.
BoxSand Introduction
Electric Potentials | Connecting the Electric Potential and Field
Connecting the Electric Potential and the Electric Field
The electric field and potential have a close relationship. In 1-D the electric field can be thought as negative the slope of the electric potential.
$\bar{E}_x = -\frac{\Delta V}{\Delta x}$
Lessons from the above equation are:
1) if $\frac{\Delta V}{\Delta x} = constant$ (linear), then $E_x$ is constant and uniform, this is the case near large sheets of charge and in parallel plate capacitors.
2) The relationship between the electric field and potential is independent of the test charge $q_0$ - it's independent of other charges interacting with the field
3) The minus sign tells us the electric field points towards decreasing electric potential - if $\frac{\Delta V}{\Delta x}$ is negative, $E_x$ is positive
In 3-D the electric field is negative the gradient of the electric potential.
$\overrightarrow{E} = - \langle \frac{\Delta V}{\Delta x}, \frac{\Delta V}{\Delta y}, \frac{\Delta V}{\Delta z} \rangle$
A gradient is a vector and can be thought of as a kind of 3-D slope that points in the direction of steepest ascent. With the minus sign the electric field points in the direction of steepest descent. Since this is the direction of the force on a positive charge we can again make the analogous connection to the gravitational field and how water is forced to run downhill, down the direction of steepest descent. This feature adds one more lesson:
4) The electric field is perpendicular to equipotential surfaces and lines
Summary
We can now go full circle with the connections between the electric field and potential, and the electric force and energy. The electric field and potential are owned by the charge distribution and preclude any interactions with new test charges $q_0$. The electric force and potential energy require an interaction with the test charge. The concept map below helps visualize the relationship.
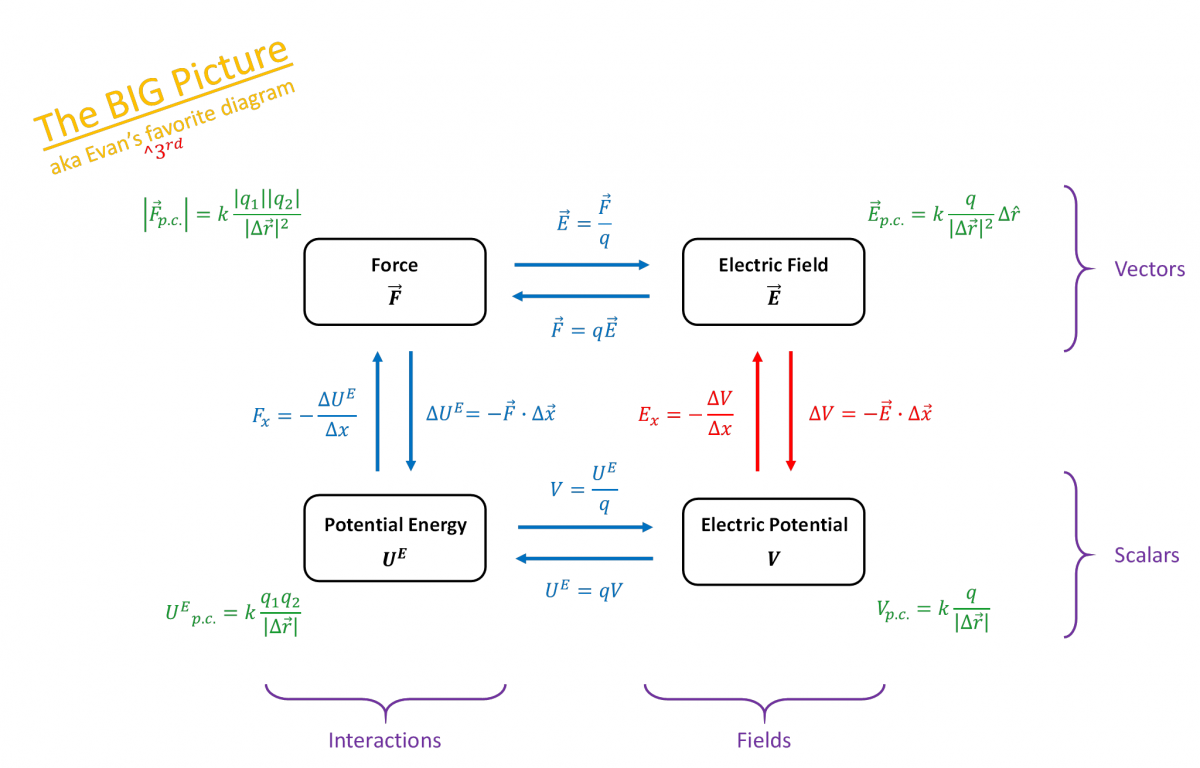
Key Equations and Infographics
Now, take a look at the pre-lecture reading and videos below.
BoxSand Videos
Required Videos
E-potential and E-field connection for uniform field(9min)
E-potential and E-field connection for nonuniform field(5min)
Suggested Supplemental Videos
OpenStax Reading
Fundamental examples
1. Two plates of metal with uniform charge distribution are held a distance d apart from each other as shown in the figure below. The metal plate on the left side is positively charged while the metal plate on the right is negatively charged.
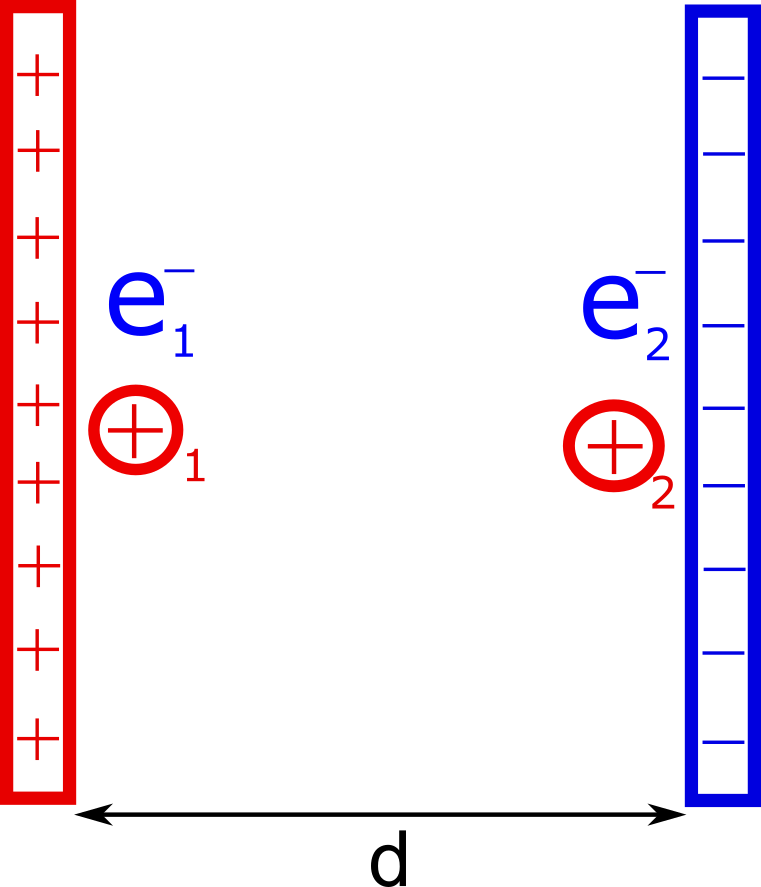
a. Which plate is considered to be at a higher potential ($V$)?
b. The electron labeled $1$ is placed in-between the two sheets as shown. Does this electron have a high or low electric potential energy? Ignore the other charges in-between the plates.
c. The electron labeled $2$ is placed in-between the two sheets as shown. Does this electron have a high or low electric potential energy? Ignore the other charges in-between the plates.
d. The proton labeled $1$ is placed in-between the two sheets as shown. Does this proton have a high or low electric potential energy? Ignore the other charges in-between the plates.
e. The proton labeled $2$ is placed in-between the two sheets as shown. Does this proton have a high or low electric potential energy? Ignore the other charges in-between the plates.
2. Two parallel plates are held $20.0 \, mm$ apart and the potential difference between the plates is $110 \, V$.
a. What is the magnitude of the electric field between the two parallel plates?
b. Which direction does the electric field point if the plate on the left has a uniform negative charge distribution and the plate on the right has a uniform positive charge distribution?
c. Sketch a physical representation of this problem. Include a few equipotential lines between the two parallel plates.
3. Two uniformly charged parallel plates are held some distance “$d$” apart from each other with an electric potential of $300 \, V$ between them. An electron is placed at rest near the negative charged plate. Calculate the speed at which the electron will hit the positive plate.
4. The figure below shows a charge distribution made up of $3$ point charges. The charges are fixed in space and cannot move. Let $q = 1 \, \mu C$ and $L = 1.0 \, cm$.
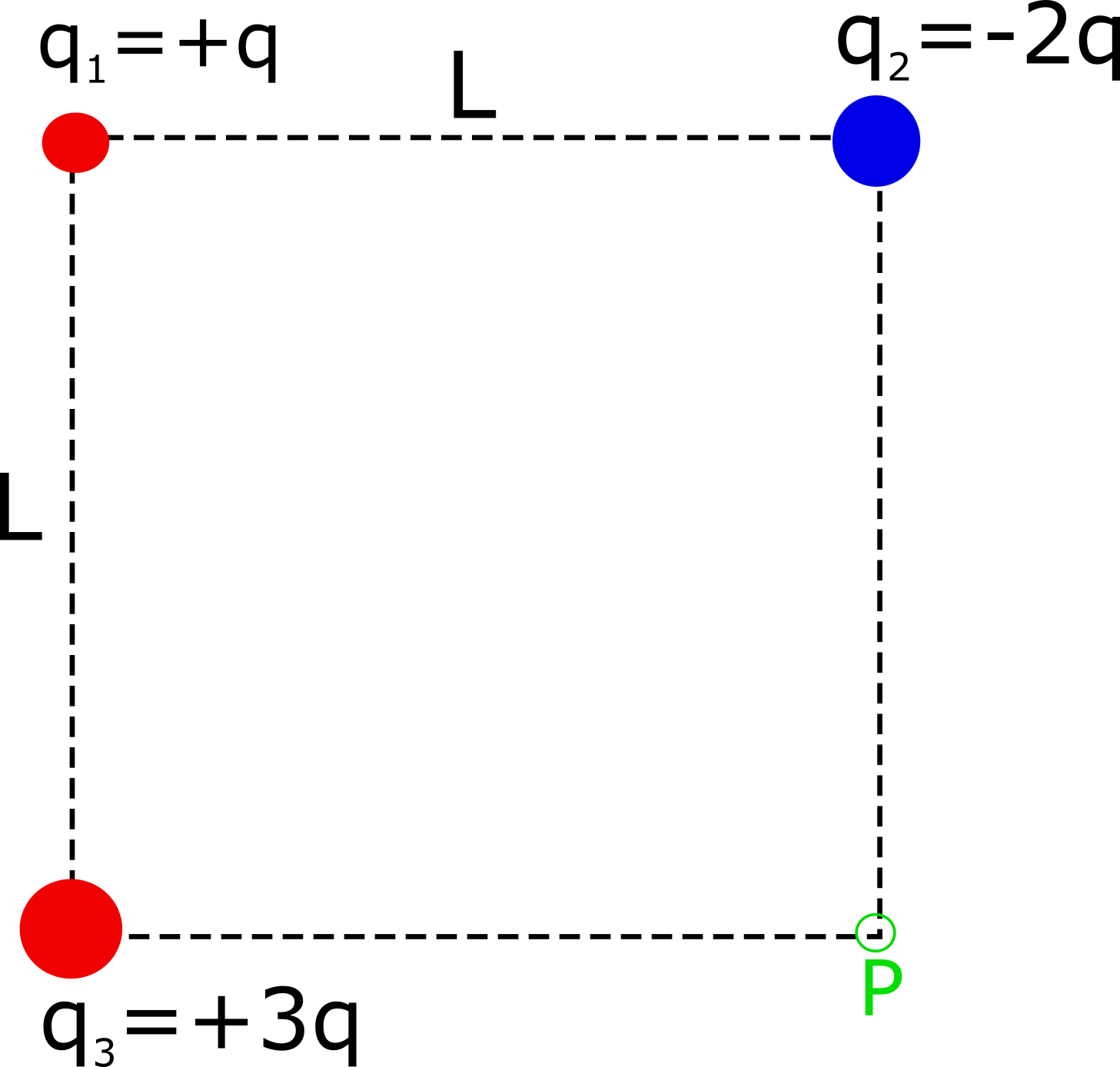
a. Find the electric potential energy of this charge distribution.
b. Find the electric potential at point P.
CLICK HERE for solutions.
Short foundation building questions, often used as clicker questions, can be found in the clicker questions repository for this subject.
Post-Lecture Study Resources
Use the supplemental resources below to support your post-lecture study.
Practice Problems
BoxSand practice problems - Answers
BoxSand's multiple select problems
BoxSand's quantitative problems
Recommended example practice problems
- OpenStax and it's 4 related sections. Problems at bottom of page,
- Electric Potential Energy, Website Link
- Electric Potential in a Uniform Electric Field, Website Link
- Electric Potential Due to a Point Charge, Website Link
- Equipotential Lines, Website Link
- Physics-Prep
- Electric Potential, Website Link
- Electric Potential Due to Point Charges, Website Link
- Equipotential Surfaces, Website Link
For additional practice problems and worked examples, visit the link below. If you've found example problems that you've used please help us out and submit them to the student contributed content section.
Additional Boxsand Study Resources
Additional BoxSand Study Resources
Learning Objectives
Summary
Summary
Atomistic Goals
Students will be able to...
YouTube Videos
Pre-Med Academy has a lot of videos about the Electric Field and Force, too many to list here. We really recommend checking out the content repository for this section and check out the rest of their videos Here are just a few on Work and Potential Energy, Electric Potential Difference, and Potential of a Point Charge
Youtube: Pre-Med Academy - Potential of a point charge
Step By Step Science has a bunch of practice problems having to do with electric potential and we suggest you check out your content repository for this section to see a list of relevant videos from them. Here are a few on work done moving a point charge through a potential difference, calculating potential difference between two points, and work to bring in a charge from infinity.
Other Resources
This link will take you to the repository of other content related resources .
Simulations
This flashphysics applet lets you create charge distributions from point charges and plot the corresponding equipotential lines. Try placing test charges in the region of space to watch possible trajectories!
This PhET interactive combines electric fields, electric potential and charge (known collectively as electrostatics). Place charges around and observe the resulting electric and potential fields.
For additional simulations on this subject, visit the simulations repository.
Demos
History
Oh no, we haven't been able to write up a history overview for this topic. If you'd like to contribute, contact the director of BoxSand, KC Walsh (walshke@oregonstate.edu).
Physics Fun
Other Resources
Resource Repository
The Physics Classroom has two sections for us, one on electric potential and the other on electric potential difference.
| Electric Potential | Electric Potential Difference |
Boston University's Page on electric potential is a neat reference with a couple of example problems
PPLATO is a complete resource with a lot of information, and several practice questions per subject. This webpage covers electric charge, the electric field, and electric potential, we've already covered charge, and the electric field, so now it's time to focus on electric potential!
Isaac Physics' section on the electric field is a good short resource. This page contains previously studied material on electric fields as well as information on electric potential, and the connection between electric field and electric potential.
Other Resources
This link will take you to the repository of other content related resources .
This link will take you to the repository of other content related resources.
Problem Solving Guide
Use the Tips and Tricks below to support your post-lecture study.
Assumptions
Checklist
Misconceptions & Mistakes
- The electric potential from a point charge is proportional to $\frac{1}{r}$, not $\frac{1}{r^2}$.
Pro Tips
Multiple Representations
Multiple Representations is the concept that a physical phenomena can be expressed in different ways.
Physical
Mathematical
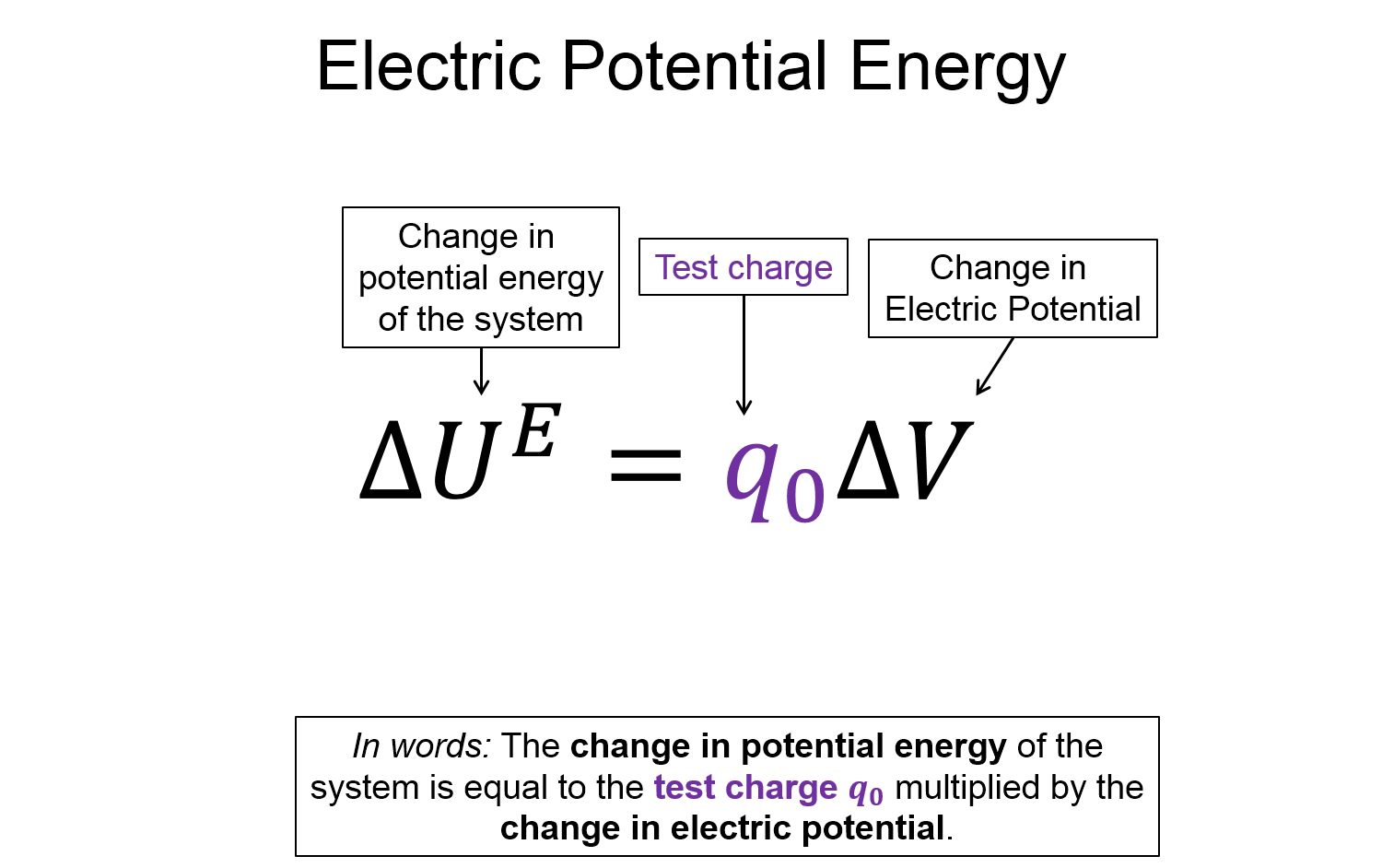
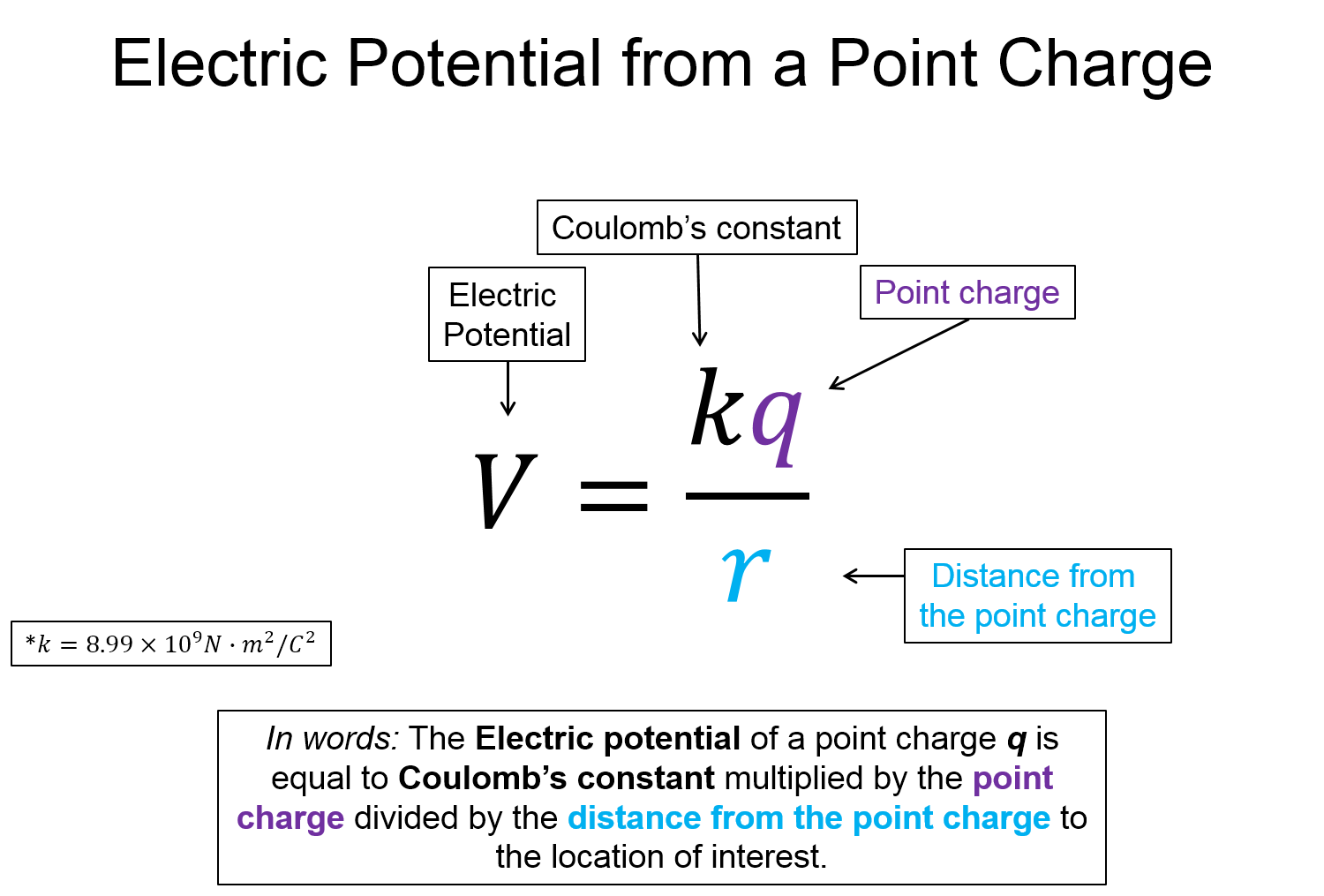

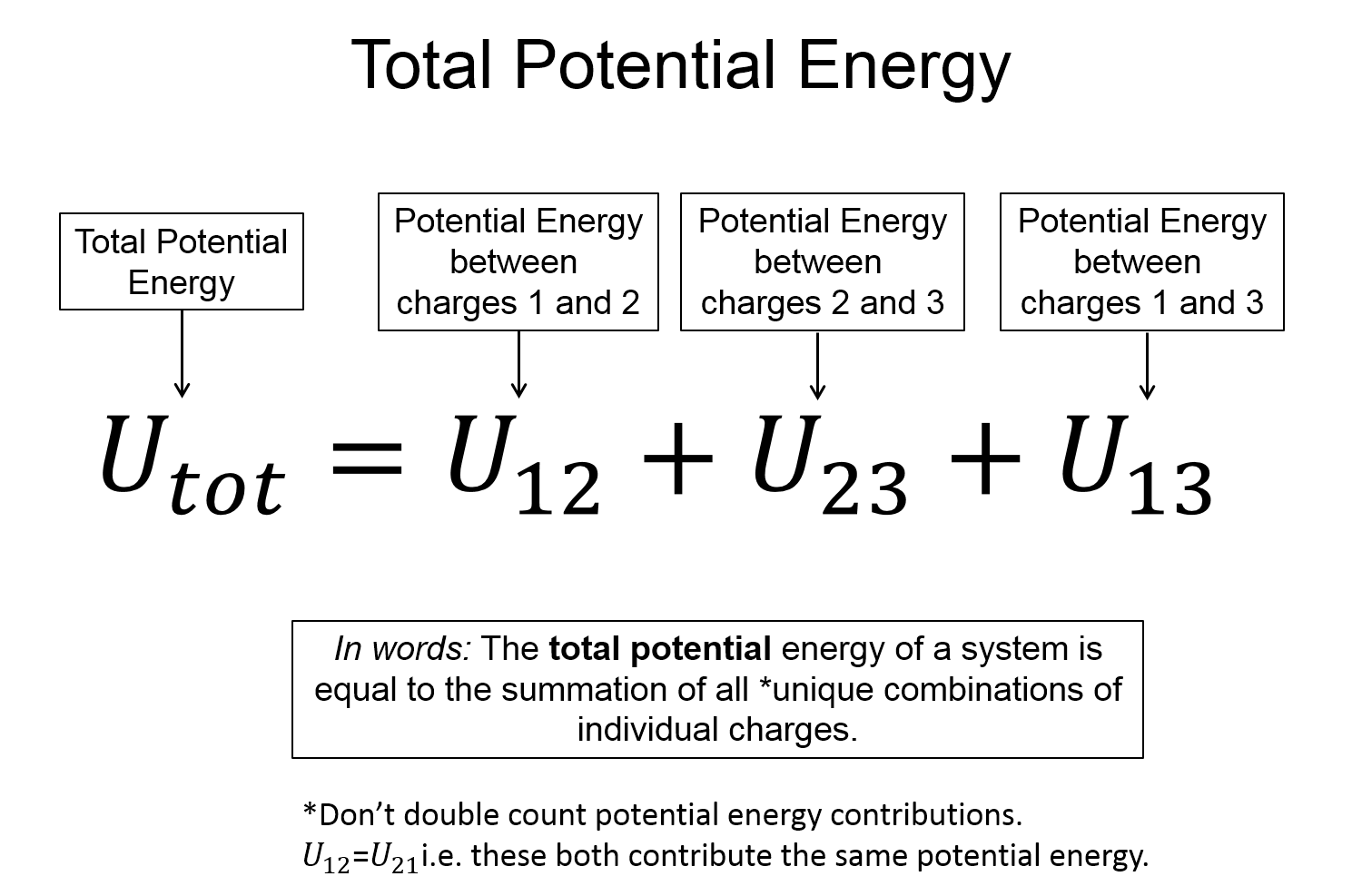
Graphical
Descriptive
Experimental