Thin lenses and spherical mirrors are very useful optical elements. They can be used to form images of objects for a variety of applications. An example would be a telescope where the goal is to magnify the image of an object located very far from the optical device. The thin lens equation allows for a mathematical solution to the features of the resulting image for a system of optical elements.
Even the Hubble Telescope needed corrective lenses to see better.
https://www.youtube.com/watch?v=E9w3MB5WiCY
Pre-lecture Study Resources
Watch the pre-lecture videos and read through the OpenStax text before doing the pre-lecture homework or attending class.
BoxSand Introduction
Ray Optics | Thin Lens Equation
Optical elements such as lenses and spherical mirrors can be used to create a variety of image features. One way to understand the effects of the optical elements is to use Ray Tracing to locate the image. While that approach is qualitatively good for understanding the features of the light rays it sometimes lacks the precision of a mathamatical model. The thin lens equation provides a mathamatical model for studying lenses, spherical mirrors, and the images they form. The figure below shows the ray diagram for a converging lens. The distance to the object is $d_o$ while the distance to the image is $d_i$. The height of the object is defined as $h_o$ while the height of the image is $h_i$. The focal point is also defined as $F$ - the focal distance, which is the distance from the optical element to the focal point, is defined as $f$.
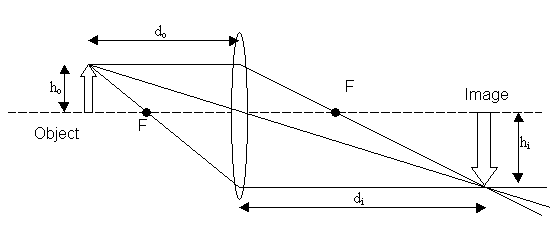
Using those variables we can relate these variables with a few equations.
Thin Lens Equation
Magnification Equation
$M=\frac{h_i}{h_o}=-\frac{d_i}{d_o}$
Use of these equations requires careful consideration to a set of sign conventions. A summary of the sign conventions are below.
Key Equations and Infographics
Now, take a look at the pre-lecture reading and videos below.
BoxSand Videos
Required Videos
Suggested Supplemental Videos
OpenStax Reading
OpenStax Section 26.1 | Physics of the Eye
OpenStax Section 26.2 | Vision Correction
Fundamental examples
Use the thin lens equation to answer all of the following problems. For all problems:
(a) Calculate the location of the image
(b) Is the image real or virtual?
(c) Calculate the magnification of the object. Is the object upright or inverted?
(1) An object is 1m from a converging lens that has a focal length of 1.5m.
(2) An object is 1m from a diverging lens that has a focal length of 1.5m.
(3) An object is 2m away from a converging lens that has a focal length of 1.5m
(4) An object is 2m away from a diverging lens that has a focal length of 1.5m.
(5) An object is 1.5m away from a converging lens that has a focal length of 1.5m.
(6) An object is 1.5m away from a diverging lens that has a focal length of 1.5m
Solutions found HERE.
Short foundation building questions, often used as clicker questions, can be found in the clicker questions repository for this subject.
Post-Lecture Study Resources
Use the supplemental resources below to support your post-lecture study.
Practice Problems
Conceptual problems
BoxSand's multiple select problems
BoxSand's quantitative problems
Recommended example practice problems
- Openstax has practice problems toward the end of each section, Website Link
For additional practice problems and worked examples, visit the link below. If you've found example problems that you've used please help us out and submit them to the student contributed content section.
Additional Boxsand Study Resources
Additional BoxSand Study Resources
Learning Objectives
Summary
Summary
Atomistic Goals
Students will be able to...
 |
 |
.png) |
 |
 |
YouTube Videos
This is a must watch introduction to the Thin Lens Equation.
https://www.youtube.com/watch?v=7GV1UZSTNJg
Khan Academy does a good job of applying the thin lens equation and how to think about solving these kinds of problems.
https://www.youtube.com/watch?v=7GV1UZSTNJg
Check out this application of thin lenses with several worked examples of mutli lens situations.
Simulations
PhET simulation for geometric optics and ray tracing
Check out this nice lens tracing simulation.
Here is a lens application with regards to the human eye.
For additional simulations on this subject, visit the simulations repository.
Demos
For additional demos involving this subject, visit the demo repository
History
Oh no, we haven't been able to write up a history overview for this topic. If you'd like to contribute, contact the director of BoxSand, KC Walsh (walshke@oregonstate.edu).
Physics Fun
Even a kitty is interested in lenses.
Other Resources
The Hyperphysics reference includes a nice 4 section discussion of the thin lens equations.
Let the people at Boundless guide you through the thin lens equation.
Resource Repository
This link will take you to the repository of other content related resources .
Problem Solving Guide
Use the Tips and Tricks below to support your post-lecture study.
Assumptions
Checklist
Misconceptions & Mistakes
Pro Tips
Multiple Representations
Multiple Representations is the concept that a physical phenomena can be expressed in different ways.
Physical
Mathematical
$\frac{1}{d_o}+\frac{1}{d_i}=\frac{1}{f}$
Graphical
Descriptive
Experimental