When light travels from one medium into another, direction of motion changes if the index of refraction of the two materials differ. Snell's Law defines how the change of direction relates to the index of refraction of the two materials.
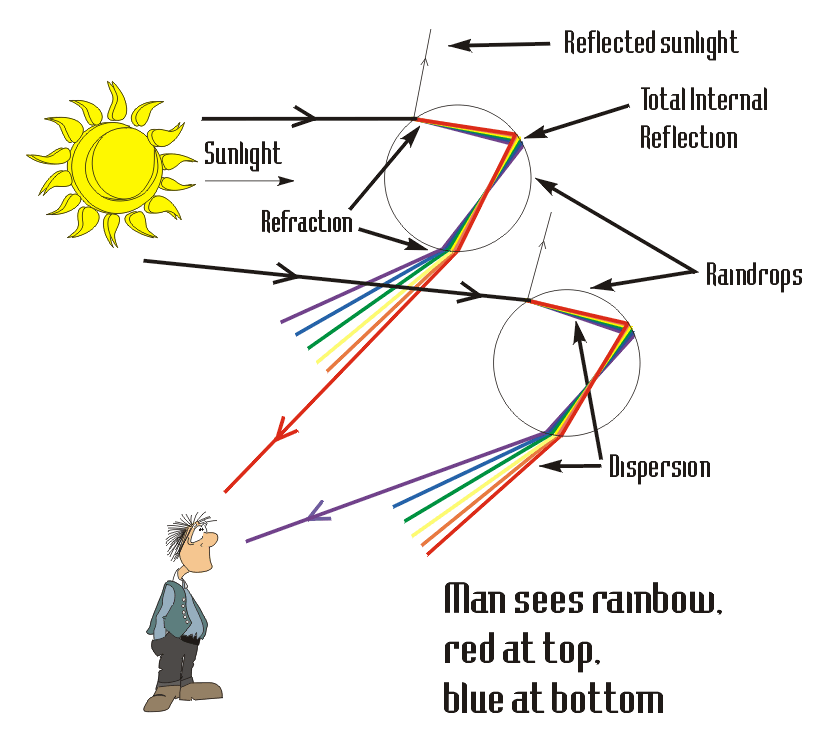
The eye uses refraction to see. Check it out!
Pre-lecture Study Resources
Watch the pre-lecture videos and read through the OpenStax text before doing the pre-lecture homework or attending class.
BoxSand Introduction
Ray Optics | Snell's Law of Diffraction
We've learned that light slows down as it enters a material with a higher index of refraction. For continuity of the wave, the frequency must remain constant. If $v=f \lambda$, and the frequency is constant but the speed decreases, the wavelength must also decrease.
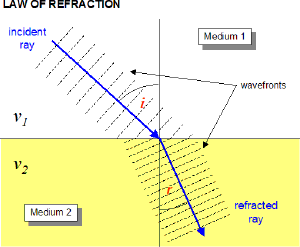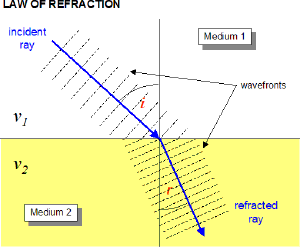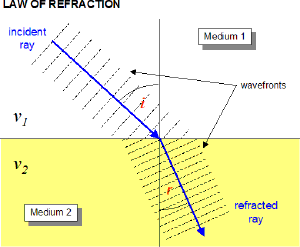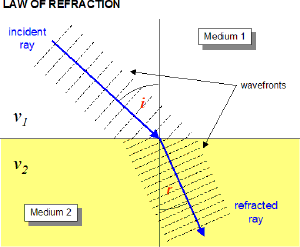
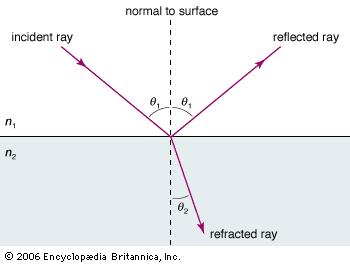
Geometrically the only way for the wavelength to decrease but the wave to remain continuous is if the angle of propagation changes. Snell's law defines the relationship between the incident angle $\theta_1$ and the refracted angle $\theta_2$ (sometimes called the transmitted angle $\theta_t$).
*NOTE: All angles are measured with respect to the normal to the surface.*
$n_1 \sin{\theta_1} = n_2 \sin{\theta_2}$
With this relationship if light travels into a medium with a higher index of refraction (transmission), like from air into water, then $\theta_1>\theta_2$ and the light bends towards the normal. If the light instead traveled in the opposite direction, so from a higher to a lower index of refraction, the light would bend away from the normal.
Total Internal Refraction (TIR)
In the right geometry, with the right relationship of index of refraction, light can actually be forced to stay within a medium and not be refracted at all. Imagine traveling from a higher to a lower index material.
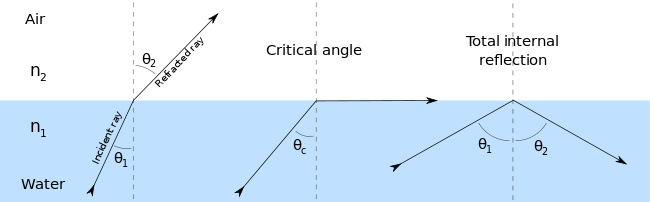
The refracted (transmitted) angle will always be larger than the incident. As $\theta_1$ increases and approaches 90º, $\theta_2$ will reach 90º before $\theta_1$ does. At the critical angle ($\theta_c$), the refracted angle is 90° and $\sin(90)=1$, so all the light is reflected and none of it is refracted.
if $n_1>n_2$, $\sin(\theta_{c}) = \frac{n_1}{n_2}$
Fiber optics, HAM radio waves that travel around the Earth, and Lightboards work on the principle of Total Internal Refraction.
Key Equations and Infographics


Now, take a look at the pre-lecture reading and videos below.
BoxSand Videos
Required Videos
Suggested Supplemental Videos
OpenStax Reading
OpenStax Section 25.3 | The Law of Refraction
OpenStax Section 25.4 | Total Internal Reflection
OpenStax Section 25.6 | Dispersion: The Rainbow and Prisms
Fundamental examples
(1) Light is traveling through air ($n = 1$) and is incident on a plastic medium at an angle of incidence of $\theta_{inc} = 40 ° $. It enters the plastic medium at an angle of inidence of $\theta_{refr} = 30 °$. What is the index of refraction of the second medium?
(2) Light travels from a medium with index of refraction $n = 2$ into a medium with an index of refraction of $n = 46$. The light crosses the boundary between mediums at an angle of $\theta =30 °$ from the horizontal. What is the angle of refraction?
(3) A piece of glass is floating on a liquid in a beaker that is open to the air. Light is incident on the piece of glass at an angle $60 °$ relative to the normal. The index of refraction of the glass is $n_g = 2.5$ and the index of refraction of the liquid is $n_l = 3$. At what angle relative to the normal does the light enter the liquid?
(4) You are standing above a glass water tank that is 1 meter deep with a red laser pointer, and you aim it down at the tank at a very shallow angle - 80 degrees from the normal. The laser exits the far wall of the tank before it hits the bottom of the tank. The horizontal distance between the laser's entry into the water and its collision with the far wall of the tank is $50$ cm. At what depth does the laser exit the tank?
Solutions found HERE.
Short foundation building questions, often used as clicker questions, can be found in the clicker questions repository for this subject.
Post-Lecture Study Resources
Use the supplemental resources below to support your post-lecture study.
Practice Problems
Conceptual problems
BoxSand's mutiple select problems
BoxSand's quantitative problems
Recommended example practice problems
- Openstax has practice problems toward the end of each section, Website Link
For additional practice problems and worked examples, visit the link below. If you've found example problems that you've used please help us out and submit them to the student contributed content section.
Additional Boxsand Study Resources
Additional BoxSand Study Resources
Learning Objectives
Summary
Summary
Atomistic Goals
Students will be able to...
YouTube Videos
Here is a good introduction to Snell's Law and its application.
Doc Schuster provides a geometric derivation of Snell's Law. This will be a very helpful video.
Here is a quick introduction to Snell's Law
Here is an apparent depth example of Snell's Law
Other Resources
This link will take you to the repository of other content related resources .
Simulations
Bending of light simulation by PhET to examine Snell's Law.
Here is an easy simulation that looks at Snell's law in an easier form.
One more Snell's law simulation to check out. This one changes the physical direction of the beam for another perspective.
For additional simulations on this subject, visit the simulations repository.
Demos
Kitty learns about refraction through experiment.
Kitty learns about refraction through experiment.
For additional demos involving this subject, visit the demo repository
History
Oh no, we haven't been able to write up a history overview for this topic. If you'd like to contribute, contact the director of BoxSand, KC Walsh (walshke@oregonstate.edu).
Physics Fun
Other Resources
This resource is very direct. Consider looking through it at least once.
The Hyperphysics reference for Snell's Law gives a quick two sentence description with a nice diagram.
Resource Repository
Other Resources
This link will take you to the repository of other content related resources .
Problem Solving Guide
Use the Tips and Tricks below to support your post-lecture study.
Assumptions
Checklist
(1) Draw a horizontal line. This represents the boundary between the two media with different indices of refraction. Draw a dotted vertical line perpendicular to the horizontal line that bisects it. This represents the line normal to the surface of the boundary between the media.
(2) Sketch the incoming ray. Remember that the angle in Snell's Law is relative to the normal, so make sure you read the problem carefully to determine if you were given that angle or an angle relative to some other line.
(3) Use Snell's Law $$n_1 sin \left( \theta_1 \right) = n_2 sin \left( \theta_2 \right) $$ and solve for the unknown varaible (you will be given some combination of 3 of the 4 variables $n_1$, $n_2$, $\theta_1$, or $\theta_2$, and asked to solve for the fourth).
More challenging Snell's Law problems will involve solving for the index of refraction or angle of refraction for some third medium. If you are given three media instead of 2, just split the problem into two stages and do the 3 steps above twice: first for $n_1$ and $n_2$, and then for $n_2$ and $n_3$ (similar to using $v_f$ from one stage of a problem as $v_i$ in the next stage).
Misconceptions & Mistakes
Pro Tips
Multiple Representations
Multiple Representations is the concept that a physical phenomena can be expressed in different ways.
Physical
Mathematical


Graphical
Descriptive
Experimental