MR-VEC-1 Which of the following vector equations correctly describes the relationship among the vectors shown in the figure?
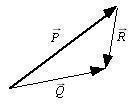
1. P + Q = R
2. P = Q + R
3. P + R = Q
4. P + Q + R =0
5. None of equations A - D is correct
MR-VEC-2 Start at the corner of a cube, of side length 1 m, and find the distance to the furthest point that still resides on the cube.
Note: the magnitude of a vector in three dimensions is the square root of the summation of the square of all three components of the vector.
Note: your answer should include units.
MR-VEC-3 You take off from La Guardia airport in a little airplane. First you fly NW for 5 miles. At the George Washington bridge, you turn SSW for 10 miles. Above the Statue of Liberty, you turn S toward the Verazzano Narrows bridge, 5 miles ahead. Over the bridge you turn ENE for a landing at JFK airport, 10 miles ahead.
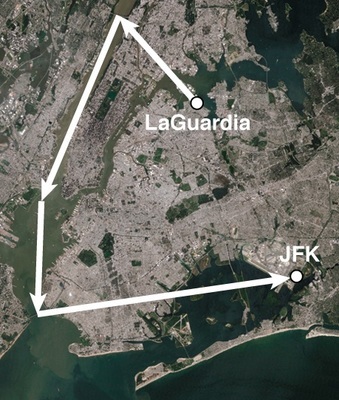
Suppose that instead of flying NW-SSW-S-ENE, you had followed the same "vectors" in a different sequence: SSW-NW-ENE-S. Mark on the map where this path would have led you.
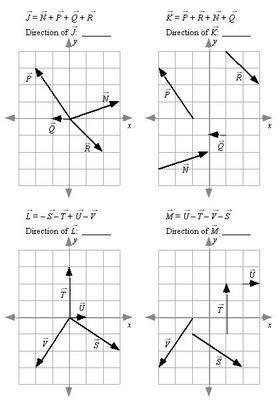
MR-VEC-4 Three vectors, labeled P, Q, and R, are shown. The length of each vector is given in arbitrary units.
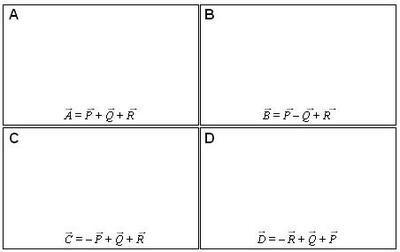
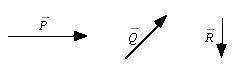
In each space provided above, construct a drawing of the indicated combinations of the vectors P, Q, and R, and then rank the magnitude of these resultant vectors.
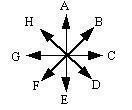
MR-VEC-5 For each situation below, combine the vectors as indicated and determine the direction of the resultant vector. Then select the closest direction to the resultant from the direction rosette.
MR-VEC-6a Estimate the position vector to the location in the room defined as point A. Set the origin at the front, bottom, right side of the room (as seen by the students). Consider left to right as the positive x direction, bottom to top as positive y direction, and front to back as the positive z direction.
Answer in whole numbers of meters with the following format: <x,y,z> m. An example might look like: <4,1,7> m.
Estimate the position vector to the location in the room defined as point B.
Using the class average for position vectors A and B, find the displacement vector from point A to B. Answer in whole numbers of meters with the following format: <Δx,Δy,Δz>. An example might look like: <4,1,7> m.
MR-VEC-6b New Origin
Estimate the position vector to the locations in the room defined as point A and point B.
This time set the origin at the front, top, left side of the room (as seen by the students). Consider left to right as the positive x direction, bottom to top as positive y direction, and front to back as the positive z direction.
Answer in whole numbers of meters with the following format: <xA,yA,zA> m, <xB,yB,zB> m. An example might look like: <4,1,7> m, <-2,3,6> m.
Using the class average for position vectors A and B from the new origin, find the displacement vector from point A to B.
Answer in whole numbers of meters with the following format: <Δx,Δy,Δz>. An example might look like: <4,1,7> m.
MR-VEC-7 Deserted on a deserted Island you spot a slightly exposed tin can under a tree. Upon opening it you find instructions to a buried treasure. It reads: “Ten paces from this very tree in a direction twenty degrees south of west lies the first location. Ten paces from this very tree in a direction sixty degrees north of east lies the second location. Walk from this tree exactly the distance and direction you would walk from the first location to the second location and you will find ye treasure. Yar” Sketch a physical representation of this situation on the grid provided. A physical representation for this type of problem should include the two position vectors, the change in the position vector, and the location of the treasure. Use the center of the grid as the tree location and scale appropriately to fit on the grid.
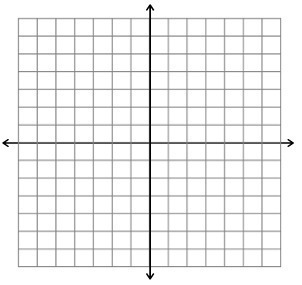
What are the coordinates of treasure?
MR-VEC-8 Upon waking from being hit in the head with a shovel you find yourself in the woods, next to a unfilled grave. You start running from this nightmare in a direction 51.34 degree from the negative y direction towards the negative x direction. Your acute direction senses surprise you but this is no time to contemplate a new super-power. You run along that line for 6.403 miles until you run into a police officer located at a location <-2, -3> miles from the police station. What are the coordinates of the grave you were presumably destined for?
1. <1, 8> mi
2. <4, -5> mi
3. <-2, 3> mi
4. <-1, -1> mi
5. <3, 1> mi